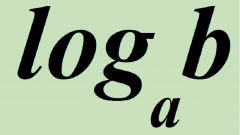How to put logarithms

The logarithm of b to base a is called the degree of x such that when raising a number a to the power x, the number of turns b: log a(b) = x ↔ a^x = b. The characteristics of the logarithms of numbers, help to reduce the addition of logarithms to multiplication of numbers.

You will need

• You need to know the properties of logarithms.

Instruction

1. Suppose you have a sum of two logarithms: the logarithm of the number b for the base a is loga(b), and the logarithm of the number d on the basis of the number of c – logc(d). This amount is written as loga(b) + logc(d).
   You may find the following solution to this problem. First, let's see whether the trivial case when the same and the base of the logarithms (a = c), and the number under the sign of the logarithm (b = d). In this case, the logarithms of the fold as a regular number or unknown. For example, x + 5 * x = 6 * x. For logarithms: 2 * log 2(8) + 3 * log 2(8) = 5 * log 2(8).
2. Next, check to see if it will work is elementary to compute the logarithm. For example, as in the following example: log 2(8) + log 5(25). Here the first logarithm is calculated as log 2(8) = log 2(2^3). I.e. to what degree it is necessary to raise the number 2 to get the number 8 = 2^3. The answer is obvious: 3. Similarly, with the following logarithm: log 5 (25) = log 5 (5^2) = 2. Thus, you will receive the sum of two natural numbers: log 2(8) + log 5(25) = 3 + 2 = 5.
3. If the base of the logarithms are equal, it is in effect a property of logarithms, known as the "log of work". According to this property the sum of logarithms with same bases is equal to the logarithm of a product: loga(b) + loga(c) = loga(bc). For example, given the amount of log 4(3) + log 4(5) = log 4(3 * 5) = log 4(15).
4. If the base of the logarithms of the sum satisfy the following expression a = c^n, you can use a property of logarithm with base a power: log a^k(b) = 1/k * log a(b). For the amount of log a(b) + log c(d) = log^n(b) + log c(d) = 1/n * log c(b) + log c(d). Therefore, the logarithms are reduced to common basis. Now you need to get rid of the factor 1/n in front of the first logarithm.
   To do this, use a property of the logarithm of the power: log a(b^p) = p * log a(b). For this example, it turns out that 1/n log c(b) = log c(b^(1/n)). Next is the multiplication property of logarithms works. 1/n * log c(b) + log c(d) = log c(b^(1/n)) + log c(d) = log c(b^(1/n) * d).
5. Use the following example for clarity. log 4(64) + log 2(8) = log 2^(1/2) (64) + log 2(8) = 1/2 log 2(64) + log 2 (8) = log 2 (64^(1/2)) + log 2(8) = log 2 (64^(1/2) * 8) = log 2 (64) = 6.
   As this example is easy to calculate, check the results: log 4(64) + log 2(8) = 3 + 3 = 6.