You will need
- - notebook;
- - the range;
- pencil;
- - handle;
- calculator.
Instruction
1
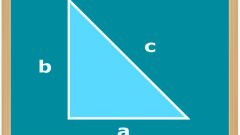
Side - side right angled triangle, forming a right angle. Opposite the right angle side of a triangle is called the hypotenuse.Since the job appears the concept "leg", we can conclude that the triangle is rectangular.
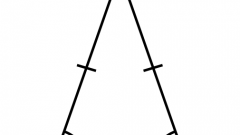
The question also says that the triangle is isosceles. This means that the legs are equal. To solve this type of task enter the legend. We denote the sides of a triangle with the letters a, a, b, where a is the legs, and hypotenuse. (see Fig. 1)
The question also says that the triangle is isosceles. This means that the legs are equal. To solve this type of task enter the legend. We denote the sides of a triangle with the letters a, a, b, where a is the legs, and hypotenuse. (see Fig. 1)
2
This:
a = a
s = 20 (a value chosen arbitrarily to illustrate the solution)Find: a
a = a
s = 20 (a value chosen arbitrarily to illustrate the solution)Find: a
3
To find the legs of isosceles triangle, use the Pythagorean theorem. It States that the square of the hypotenuse of a rectangular triangle is equal to the sum of the squares of the other two sides. Formula: a^2+at^2=s^2.
4
The solution:a^2+a^2=C^2
2A^2=C2 (this conversion happened because in our specific task, both sides are equal)
Substitute the known data:
2A^2=400 (400 is the square of the hypotenuse)
a^2=200 (both parts of the equation are divided into two)
a=√200 10√2Ответ: √200
2A^2=C2 (this conversion happened because in our specific task, both sides are equal)
Substitute the known data:
2A^2=400 (400 is the square of the hypotenuse)
a^2=200 (both parts of the equation are divided into two)
a=√200 10√2Ответ: √200
Note
The legs exist only in a right triangle.
Useful advice
Verna theorem and inverse theorem of Pythagoras: if the square side of the triangle is equal to the sum of the squares of the other two sides, then the triangle is rectangular.