Instruction
1
Two sides and angle between them
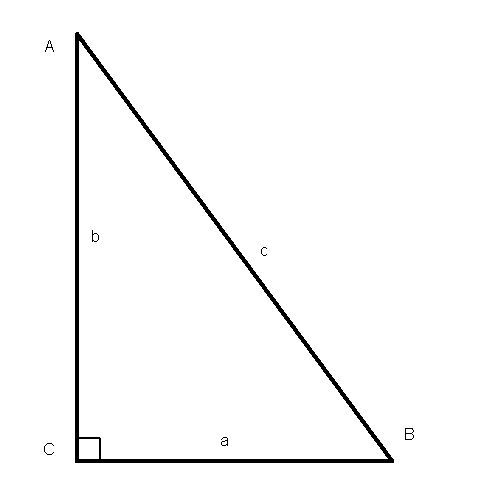
If you know the lengths of two sides of a triangle and the angle between them, then to find the length of the third side using the theorem of cosines: the square of the length of the sides of a triangle equals the sum of the squares of the lengths of its two other sides minus twice the product of these sides into the cosine of the angle between them.
Hence we have:
C=√(A2+b2-2аb*cosC), where
a and b are the lengths of the known sides
With the size of the angle between these sides (opposite the side)
– the length of the required side.
Example 1.
Given a triangle with sides of 10 cm and 20 cm and the angle between them is 60 degrees. Find the length of a side.
Solution.
According to the above formula we get:
with=√(102+202-2*10*20*cos60º)=√(500-200)=√300~17,32
Answer: the length of a side of the triangle opposite the sides with lengths 10 and 20 cm and the size of the angle between them is 60º - ~17,32 see
If you know the lengths of two sides of a triangle and the angle between them, then to find the length of the third side using the theorem of cosines: the square of the length of the sides of a triangle equals the sum of the squares of the lengths of its two other sides minus twice the product of these sides into the cosine of the angle between them.
Hence we have:
C=√(A2+b2-2аb*cosC), where
a and b are the lengths of the known sides
With the size of the angle between these sides (opposite the side)
– the length of the required side.
Example 1.
Given a triangle with sides of 10 cm and 20 cm and the angle between them is 60 degrees. Find the length of a side.
Solution.
According to the above formula we get:
with=√(102+202-2*10*20*cos60º)=√(500-200)=√300~17,32
Answer: the length of a side of the triangle opposite the sides with lengths 10 and 20 cm and the size of the angle between them is 60º - ~17,32 see
2
According to two angles and the side
If the known values of two angles and the length of one of the sides of a triangle, the lengths of the other two sides the easiest to find using the theorem of sines: the ratio of the sines of the angles of a triangle to the lengths of opposite sides are equal.
sinA/a=sinB/b=sinC/C where:
a, b, c the lengths of the sides of a triangle, and A, B, C – values of the opposite corners.
What are the angles of a triangle is known – is not important, since, using the fact that the sum of the angles of a triangle equal 180 degrees, you can easily find out the value of the unknown angle.
That is, for example, if the known values of angles A and C and side length a, side length s will be:
C=a*sinC/sinA
If the known values of two angles and the length of one of the sides of a triangle, the lengths of the other two sides the easiest to find using the theorem of sines: the ratio of the sines of the angles of a triangle to the lengths of opposite sides are equal.
sinA/a=sinB/b=sinC/C where:
a, b, c the lengths of the sides of a triangle, and A, B, C – values of the opposite corners.
What are the angles of a triangle is known – is not important, since, using the fact that the sum of the angles of a triangle equal 180 degrees, you can easily find out the value of the unknown angle.
That is, for example, if the known values of angles A and C and side length a, side length s will be:
C=a*sinC/sinA
3
If the same source data, you need to find the length of side b, then to use the theorem of sines, we need to know the size of this angle:
since B=180º-A-C, the length of side b can be found by the formula:
b=a*sin(180º-A-C)/sinA
Example 2.
Let in triangle ABC the known side length a=10 cm and angles A=30 and C=20. To find the length of side b.
Solution: in the above formula we get:
b=10*sin(180º-30º-20º)/sin30º=10*sin130º/0,5=5*sin130º~3,83
Answer: the length of a side of a triangle is ~3,83 see
since B=180º-A-C, the length of side b can be found by the formula:
b=a*sin(180º-A-C)/sinA
Example 2.
Let in triangle ABC the known side length a=10 cm and angles A=30 and C=20. To find the length of side b.
Solution: in the above formula we get:
b=10*sin(180º-30º-20º)/sin30º=10*sin130º/0,5=5*sin130º~3,83
Answer: the length of a side of a triangle is ~3,83 see