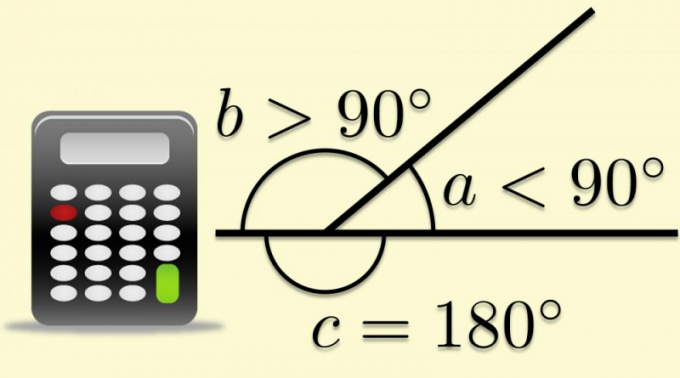How to find the cosine of the external angle

Any angle can be extended to the deployed, if to extend over the top of one of its sides. While the other side will divide a straight angle into two. The angle formed by the second party and the first called adjacent, and when we are talking about polygons, so it called external. The fact that the sum of external and internal angles, by definition, equal to the value of the maximized angle allows you to calculate trigonometric functions on the known ratios of parameters of the polygons.

How to find the cosine of the external angle
How to find the cosine if you know the sine
How to find angles when you know the lengths of the sides of a triangle
As the cosine to find a side of a triangle

Instruction

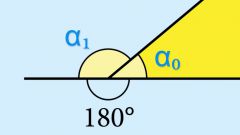
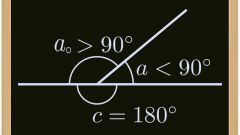
Knowing the result of the computation of the cosine of the internal angle (α) you'll know the module of the cosine external (α₀). The only thing that you need to produce with this value to change its sign, i.e., multiplied by -1: cos(α₀) = -1*cos(α).

If we know the value of the internal angle (α) to calculate the cosine of an external (α₀) you can use the method described in the previous step is to find its cosine, and then to change the sign. But it can be done differently - just compute the cosine of the external angle, subtracting this value from the inner 180°: cos(α₀) = cos(180°-α). If the magnitude of the internal angle is given in radians, the formula needs to be converted to this: cos(α₀) = cos(π-α).

The regular polygon to calculate the value of the external angle (α₀) does not need to know any parameters except the number of vertices (n) of this figure. This number divide 360° find the cosine of the resulting numbers: cos(α₀) = cos(360°/n). For calculations in radians on the number of vertices you have to share twice the number PI, and the formula should acquire the following form: cos(α₀) = cos(2*π/n).

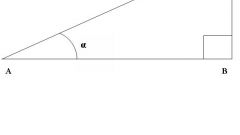
In a right triangle, the cosine of the external angle at the vertex lying opposite the hypotenuse is always equal to zero. For the other two vertices, this value can be calculated, knowing the length of the hypotenuse (c) leg (a), which form the top. No trigonometric functions to calculate required, just divide the length of the short side length of the larger, and change the sign of the result is: cos(α₀) = -a/c.

If you know the lengths of two sides (a and b), too, can do the calculations without trigonometric functions, but the formula is somewhat more complicated. Fraction, the denominator of which is the length of the side adjacent to the top outer corner, and in the numerator - the length of the other leg defines the tangent of the internal angle. Knowing the tangent, you can calculate the cosine of the internal angle: √(1/(1+a2/b2). This expression will replace the cosine in the right part of the formula from the first step: cos(α₀) = -1*√(1/(1+a2/b2).

Is the advice useful?