Instruction
1
Imagine that some point A for a certain period of time t has passed a way's. The equation of motion of point A can be written in the following form:
s=f(t), where f(t) is a function of distance traveled
Because the speed is found by dividing the path for a while, it is a derivative way, and, accordingly, the above-mentioned functions:
v=s t=f(t)
When you change the speed and time speed is calculated as follows:
v=Δs/Δt=ds/dt=s t
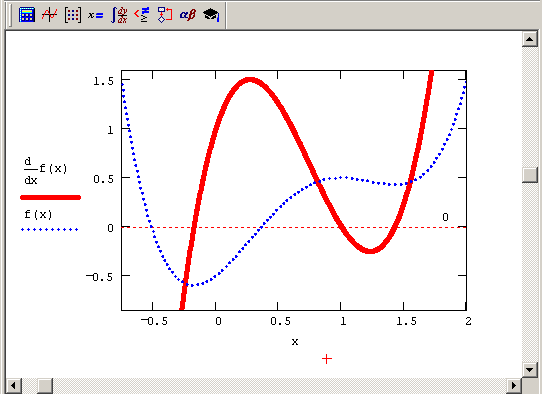
All values of velocity is derivative of the way. For a certain period of time, respectively, can change and speed. In addition, the method of differential calculus to find the acceleration, which is the first derivative of velocity and second derivative of the path. When we speak of the second derivative of the function, we are talking about the differentials of the second order.
s=f(t), where f(t) is a function of distance traveled
Because the speed is found by dividing the path for a while, it is a derivative way, and, accordingly, the above-mentioned functions:
v=s t=f(t)
When you change the speed and time speed is calculated as follows:
v=Δs/Δt=ds/dt=s t
All values of velocity is derivative of the way. For a certain period of time, respectively, can change and speed. In addition, the method of differential calculus to find the acceleration, which is the first derivative of velocity and second derivative of the path. When we speak of the second derivative of the function, we are talking about the differentials of the second order.
2
Differential of a function from a mathematical point of view is a derivative that is written in the following form:
dy=df(x)=y dx=f'(x)Δx
When given an ordinary function, expressed in numeric values, the differential is calculated by the following formula:
f'(x)=(x^n)'=n*x^n-1
For example, in the problem given a function f(x)=x^4. Then the differential of this function is equal to: dy =f'(x) =(x^4)'=4x^3
Simple differentials of trigonometric functions is given in all reference books on higher mathematics. The derivative of the function y=sin x is equal to the expression (y)'=(sinx)'=cosx. Also reference the differentials of a number of logarithmic functions.
dy=df(x)=y dx=f'(x)Δx
When given an ordinary function, expressed in numeric values, the differential is calculated by the following formula:
f'(x)=(x^n)'=n*x^n-1
For example, in the problem given a function f(x)=x^4. Then the differential of this function is equal to: dy =f'(x) =(x^4)'=4x^3
Simple differentials of trigonometric functions is given in all reference books on higher mathematics. The derivative of the function y=sin x is equal to the expression (y)'=(sinx)'=cosx. Also reference the differentials of a number of logarithmic functions.
3
Differentials of complex functions are calculated by using the table of differentials and the knowledge of some of their properties. The following are the basic properties of the differential.
Property 1. Differential of a sum is the sum of the differentials.
d(a+b)=da+db
This property applies no matter what function was given trigonometric or ordinary.
Property 2. The constant factor can be taken out of the differential sign.
d(2a)=2d(a)
Property 3. The product of the complex differential of the function is equal to the product of one simple function on the differential of the second, folded with a second work function on the differential first. It looks as follows:
d(uv)=du*v+dv*u
Such an example is the function y=x sinx, the differential of which is equal to:
y'=(xsinx)'=(x) x*sinx+(sinx)'*x=sinx+cosx^2
Property 1. Differential of a sum is the sum of the differentials.
d(a+b)=da+db
This property applies no matter what function was given trigonometric or ordinary.
Property 2. The constant factor can be taken out of the differential sign.
d(2a)=2d(a)
Property 3. The product of the complex differential of the function is equal to the product of one simple function on the differential of the second, folded with a second work function on the differential first. It looks as follows:
d(uv)=du*v+dv*u
Such an example is the function y=x sinx, the differential of which is equal to:
y'=(xsinx)'=(x) x*sinx+(sinx)'*x=sinx+cosx^2