Instruction
1
Reference material.
To begin with we define a tangent. Tangent to the curve at a given point M is called the limiting position of the secant NM, when the point N along the curve is approaching to the point M.
Find the equation of the tangent to the graph of the function y = f(x).
To begin with we define a tangent. Tangent to the curve at a given point M is called the limiting position of the secant NM, when the point N along the curve is approaching to the point M.
Find the equation of the tangent to the graph of the function y = f(x).
2
Determined by the slope of the tangent to the curve at the point M.
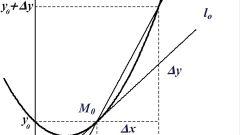
The curve representing the graph of the function y = f(x), continuous in some neighborhood of the point M (including the M).
Spend section MN1, forming with the positive direction of the Ox axis the angle α.
The coordinates of the point M (x; y), the coordinates of a point N1(x+∆x, y+∆y).
From the resulting triangle MN1N you can find the slope of this secant:
tg α = Δy/Δx
MN = ∆x
NN1 = ∆y
As the point N1 on the curve the point M to the secant MN1 turns around a point M, and the angle α tends to the angle ϕ between the tangent MT and the positive direction of the Ox axis.
k = tg ϕ =〖 lim〗┬(∆x→0)〖 〗 Δy/Δx = f`(x)
Thus, the slope of the tangent to the graph of the function is equal to the value of the derivative of this function at the tangent point. This is the geometric meaning of the derivative.
The curve representing the graph of the function y = f(x), continuous in some neighborhood of the point M (including the M).
Spend section MN1, forming with the positive direction of the Ox axis the angle α.
The coordinates of the point M (x; y), the coordinates of a point N1(x+∆x, y+∆y).
From the resulting triangle MN1N you can find the slope of this secant:
tg α = Δy/Δx
MN = ∆x
NN1 = ∆y
As the point N1 on the curve the point M to the secant MN1 turns around a point M, and the angle α tends to the angle ϕ between the tangent MT and the positive direction of the Ox axis.
k = tg ϕ =〖 lim〗┬(∆x→0)〖 〗 Δy/Δx = f`(x)
Thus, the slope of the tangent to the graph of the function is equal to the value of the derivative of this function at the tangent point. This is the geometric meaning of the derivative.
3
The equation of the tangent to a given curve at a given point M has the form:
y - y0 = f`(x0) (x - x0),
where (x0; y0) is the coordinate of the touch point
(x; y) the current coordinates, i.e. the coordinates of any point belonging to the tangent,
f`(x0) = k = tg α is the slope of the tangent.
y - y0 = f`(x0) (x - x0),
where (x0; y0) is the coordinate of the touch point
(x; y) the current coordinates, i.e. the coordinates of any point belonging to the tangent,
f`(x0) = k = tg α is the slope of the tangent.
4
Find the equation of the tangent, for example.
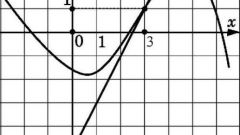
Given the graph of the function y=x2 – 2x. Need to find the equation of the tangent at the point with abscissa x0 = 3.
From the equation of the given curve find the ordinate of the touch point y0 = 32 - 2∙3 = 3.
Find the derivative and then calculate its value at the point x0 = 3.
Have:
y`=2x – 2
f`(3) = 2∙3 – 2 = 4.
Now that we know the point (3; 3) on the curve and the angular factor f`(3) = 4 the tangent at that point, we get the desired equation:
y – 3 = 4 (x – 3)
or
y – 4x + 9 = 0
Given the graph of the function y=x2 – 2x. Need to find the equation of the tangent at the point with abscissa x0 = 3.
From the equation of the given curve find the ordinate of the touch point y0 = 32 - 2∙3 = 3.
Find the derivative and then calculate its value at the point x0 = 3.
Have:
y`=2x – 2
f`(3) = 2∙3 – 2 = 4.
Now that we know the point (3; 3) on the curve and the angular factor f`(3) = 4 the tangent at that point, we get the desired equation:
y – 3 = 4 (x – 3)
or
y – 4x + 9 = 0