Instruction
1
In order to divide common fraction to common fraction, multiply the first fraction by the inverted second fraction. This "inverted" ordinary fraction, where the numerator and denominator are reversed is called reverse.
When dividing fractions it is necessary to pay attention to the fact that the second fraction is not equal to zero. Sometimes, if the fraction is quite bulky, it is extremely difficult to make. In addition, the second fraction can contain some variables (unknown) value, which for certain values of the draw roll to zero. You also need to pay attention to those cases where the denominator of the second fraction vanishes. When the action variables for all these cases, you must specify in the final answer.
For example: see Fig. 1
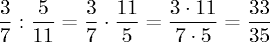
2
To divide a mixed fraction by a mixed, a mixed fraction to an ordinary or common in mixed, get mixed fractions to an ordinary mind. Then to make the division as specified in step 1.
To transfer mixed fraction to an ordinary need the integer part of mixed fraction multiplied by its denominator and add the resulting product to the numerator.
Example: see Fig. 2
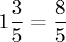
3
When dividing decimal fractions to ordinary (mixed) or division of ordinary (mixed) fraction to a decimal, all fractions are reduced to the ordinary mind. After this division is done according to step 1. To translate decimals to fractions, the "throw" of the decimal point and recorded in the numerator and in the denominator write one and as many zeros as digits stood to the right of the decimal point.
Example: see Fig. 3
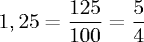
4
To divide two decimals need in divisible and the divider to move the decimal point that many digits to the right of the second fraction to make a whole number and divide the resulting number.
For example: 24,68/123,4=246,8/1234=0,2.
If the divisible to transfer the decimal point in the "missing" digits, missing digits are replaced with zeros.
Example: 24,68/1,234=24680/1234=20
For example: 24,68/123,4=246,8/1234=0,2.
If the divisible to transfer the decimal point in the "missing" digits, missing digits are replaced with zeros.
Example: 24,68/1,234=24680/1234=20
Note
The divisor and denominator of a fraction can't be 0.