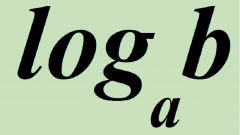How to find the log base

By definition of the logarithm the result of his calculation is the measure of the degree to which it is necessary to build a base. On this basis, to calculate the base are inverse operation of exponentiation, that is, remove the root. If the base is denoted by x, podogretuju variable through a, and the value of the logarithm of a in base x is in n, then the identities logₓa = n implies the identity x = ⁿ√a.

From the previous step it follows that to calculate the unknown base of a logarithm you need to know the number from which was extracted the logarithm, and the result of this operation. For example, if the original number was 729, and the logarithm of it is equal to six, to calculate the base logarithm of 729 remove the root of the sixth degree: ⁶√729 = 3. Conclusion: base of the logarithm equals three.

For practical calculations when finding the base of the logarithm it is convenient to use the calculator built into Google search. For example, knowing what a logarithm was extracted from the number 14641, and the result of this operation is equal to four, go to the home page of a search engine and type in the text box only a query: 14641^(1/4). Here "cover" ^ means the operation of exponentiation, a decimal figure in parentheses causes the calculator engine to produce the reverse operations of root extraction. After sending a request to the Google server will perform the calculations and determine the desired index of the logarithm: 14 641^(1 / 4) = 11.

The same can be done with the built-in operating system of the calculator. In the latest versions of OS for his call, just press Win, type "ka" and press Enter. The desired function of the root placed in the "engineering" option of the program is to use Alt + 2 to enable it. For example, from the previous step, you must enter the number 14641, click the button with the symbol ʸ√x, enter 4 and press Enter. The result is the same (11).