You will need
  • mathematical Handbook;
  • - the range;
  • - pencil;
  • - notebook;
  • - handle.
Instruction
1
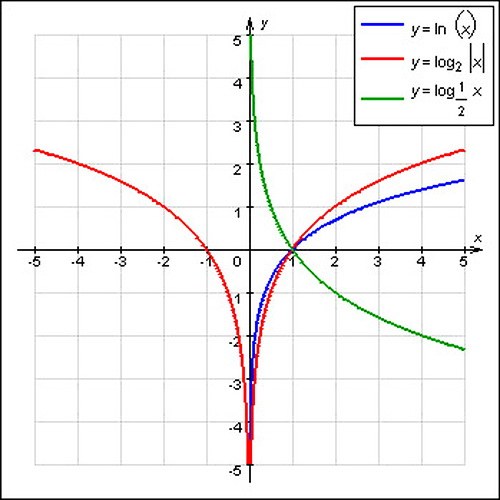
Before you can begin to build the graph of the logarithmic function notice that the domain of definition of this function is the set of positive numbers: this value is denoted by R+. However, the logarithmic function is the domain of values, which is represented by real numbers.
2
Carefully read the conditions of the job. If a>1, the flow chart depicted an increasing logarithmic function. To prove this feature of the logarithm function easy. For example, take two arbitrary positive values of x1 and x2, and x2>x1. Prove that loga x2>x1 loga (this can be done by contradiction).
3
Assume that loga x2≤loga x1. Given that the exponential function of the form y=Ah if set and>1 increases, the inequality will be as follows: aloga x2≤x1 aloga. On the known definition of the logarithm aloga x2=x2, while aloga x1=x1. Because of this, the inequality becomes: x2≤x1, and this is directly contrary to initial assumptions, in accord with which x2>x1. So, you have come to the conclusion that we wanted to prove: if a>1 the logarithmic function is increasing.
4
Draw the graph of the logarithmic function. The graph of the function y = logax will pass through the point (1;0). If a>1, the function will be increasing. Therefore, if 0