Instruction
1
In the General case, when finding an inverse function for a given y = f(x) to Express the argument x in the function. Use the rules of multiplying both parts of equality on the same value, the transfer of polynomial expressions, in this case, consider sign changes. In a simple case, the consideration of exponential functions of the form: y = (7/x) + 11, case, argument x is simple: 7/x = -11 x = 7*(y-11). The desired inverse function is x = 7*(y-11).
2
Often, however, the functions are complex exponential and logarithmic expressions, and trigonometric functions. In this case, when finding the inverse functions need to take into account the known properties of these mathematical expressions.
3
If the original function argument x is under the degree, to obtain the inverse functions take the expression from the root to the same index. For example, for a given function y = 7+ x2 inverse will be of the form: f(y) = √at -7.
4
When considering functions where the argument x represents the degree of a constant number, use the definition of logarithm. From this it follows that for the function f(x) = Ah inverse will be f(u) = logаy, and the base of the logarithm and in both cases, a number other than zero. Similarly and conversely, considering the source of the logarithmic function f(x) = logах, its inverse function is a power-law expression: f(u) = AU.
5
In the particular case of the study of functions containing the natural logarithm ln x or lg x decimal, i.e. logarithms to base e and 10 respectively receive inverse functions is similar, only instead of ground and substituted the exponential number or the number 10. For example, f(x) = lg x -> f(u) = 10U and f(x) = ln x -> f(y) = EY.
6
For trigonometric functions are inverse to each other are the following pairs:
- y = cos x -> x = y asso;
- y = sin x -> x = y agsp;
- y = tan x -> x = y аrctan.
- y = cos x -> x = y asso;
- y = sin x -> x = y agsp;
- y = tan x -> x = y аrctan.
Note
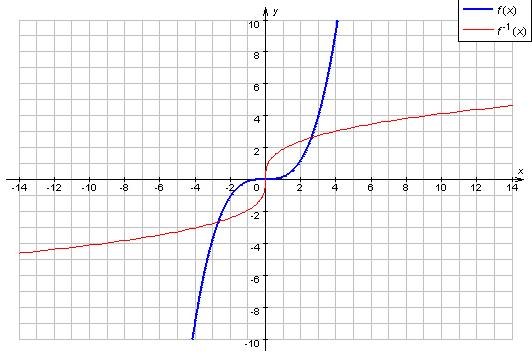
It should be remembered that a continuous function can be reversed only on the intervals of its values, where it is monotonic.