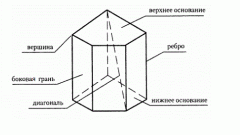
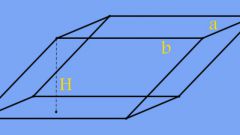
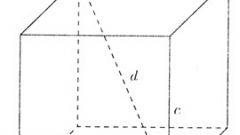
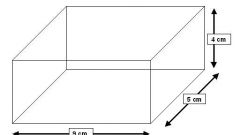
Euclid, Greek mathematician and the founder of elementary geometry, gave this definition of a prism - a solid figure enclosed by two equal and parallel planes (the bases) and lateral faces parallelograms. In ancient mathematics there was no concept of a limited part of the plane, the scientist meant by "bodily shape." Thus, the prism, as well as any other geometrical figure, is not empty.A few basic definitions: • lateral surface – the totality of all the lateral faces. • full surface – the set of all faces (bases and lateral surface); • altitude – line segment perpendicular to the bases of the prism and connecting them; • diagonal – a segment connecting two vertices of the prism that do not belong to the same face; • the diagonal plane is the plane passing through the diagonal of the base prism and its lateral edge; • diagonal cross-section is a parallelogram obtained by the intersection of a prism and a diagonal plane. Special cases of the diagonal of the cross section: rectangle, square, rhombus; • perpendicular to the cross section is a plane passing perpendicular to the side edges.The basic properties of a prism • base of a prism is equal and parallel polygons; • the lateral faces of a prism are always parallelograms; • the lateral edges of the prism are parallel to each other and have equal length.Distinguish between straight and angled right prism: • a right prism, all lateral edges are perpendicular to the base; • oblique prism lateral edges aperpendicular base; • right prism – a polyhedron with a regular polygon at the base and lateral edges are perpendicular to the bases. The right prism is a straight line.Basic numerical characteristics of prisms: • the volume of a prism equals the area of the base in height; • the lateral surface area is the product of the perimeter of the cross section perpendicular to the length of a side edge; • the total surface area of a prism is the sum of all of the areas of the lateral faces and the area of the base multiplied by two.
What is prism
Prism - a geometric shape, a polyhedron with two equal and parallel faces, called bases and having the shape of a polygon. The other faces are the bases of their sides are called lateral.
Is the advice useful?