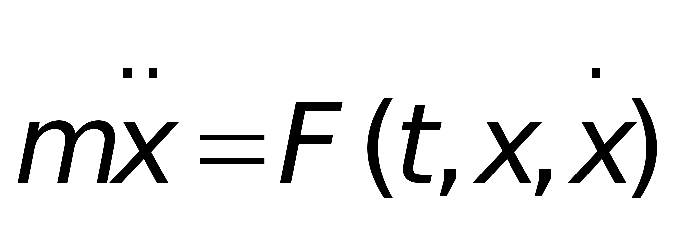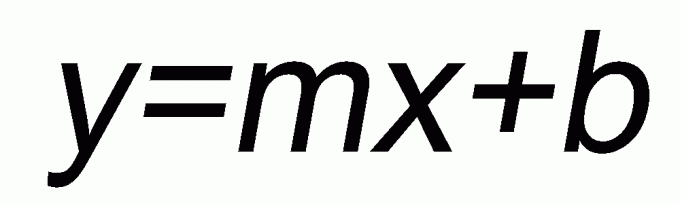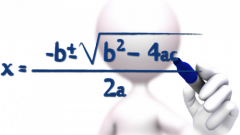Instruction
1
In the most General and simple classification of the equation can be divided by the number of variables they contain and the degree in which these variables are.
To solve an equation means to find all its roots or to prove that they are not.
Any of the equations has at most P roots, where P is the maximum degree of a given equation.
But some of these roots may coincide. For example, the equation x^2+2*x+1=0, where ^ is the icon exponentiation, folded in a square of expression (x+1), that is the product of two identical brackets, each of which gives x=-1 as solutions.
To solve an equation means to find all its roots or to prove that they are not.
Any of the equations has at most P roots, where P is the maximum degree of a given equation.
But some of these roots may coincide. For example, the equation x^2+2*x+1=0, where ^ is the icon exponentiation, folded in a square of expression (x+1), that is the product of two identical brackets, each of which gives x=-1 as solutions.
2
If the equation has only one unknown, it means that you will be able to explicitly find the roots (real or complex).
To do this, most likely need different transformations: formulas of reduced multiplication to the calculation formula of the discriminant and roots of a quadratic equation, the transfer of terms from one part to another, the bringing to a common denominator, multiply both sides by the same expression, squaring, etc.
Conversion, not affecting the roots of the equation are called identical. They are used to simplify the solution of the equation.
You can also instead of the traditional use of analytical graphical method and write the given equation as a function, then conducting her research.
To do this, most likely need different transformations: formulas of reduced multiplication to the calculation formula of the discriminant and roots of a quadratic equation, the transfer of terms from one part to another, the bringing to a common denominator, multiply both sides by the same expression, squaring, etc.
Conversion, not affecting the roots of the equation are called identical. They are used to simplify the solution of the equation.
You can also instead of the traditional use of analytical graphical method and write the given equation as a function, then conducting her research.
3
If the equation more than one unknown, you can only Express one of them through the other, thereby showing the set of solutions. Such, for example, equations with parameters, which contain the unknown x and the parameter a. To solve the parametric equation means for all and to Express x in a, that is, to consider all possible cases.
If in the equation are derivatives or differentials of an unknown (see picture), congratulations, it's a differential equation, and then you can not do without mathematics).
If in the equation are derivatives or differentials of an unknown (see picture), congratulations, it's a differential equation, and then you can not do without mathematics).