How to find the height of the right pyramid

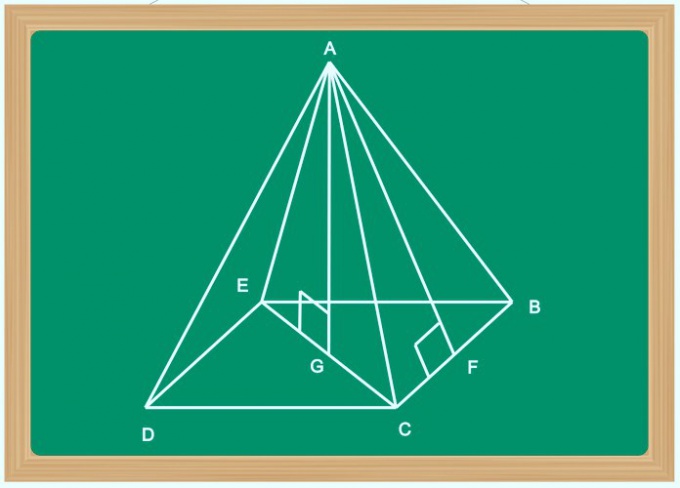
If the conditions of the problem given the area of the Foundation (s) and volume (V) of a pyramid, the formula for calculating the height of the polytope (H) is very simple - divide triple the volume of the square: H = 3*V/s.

When the square base of the pyramid with the known length of a side (a) and a given volume (V), replace the square in the formula from the previous step into the squared side length: H = 3*V/a2.

The formula of the first step can be transformed to calculate the height (H) of a regular pyramid c base of any shape. The source data that it needs to be activated - volume (V) of the polyhedron the edge length in the base (a) and the number of vertices at the base (n). Area of a regular polygon is determined by the quarter of the works by the number of vertices on the square of the length of the sides and the cotangent of an angle is equal to the ratio of 180° and the number of vertices: ¼*n*a2*ctg(180°/n). Substitute this expression into the formula from the first step: H = 3*V/(¼*n*a2*ctg(180°/n)) = 12*V/(n*a2*ctg(180°/n)).

Apofema (h) any of the lateral faces regular pyramid forms with a radius inscribed in the base circle (r) and the height of a regular pyramid (H) right triangle. If the radius and apofema known use in the calculation of the Pythagorean theorem. As the required value here is the side of the theorem imply that you need to take the square root of the difference between the square of apogamy (hypotenuse) and the radius squared (second leg): H = √(h2-r2).

At a certain apofema (h) and tilt angle (α) side face to the base of a regular pyramid in the calculation formula of height (H), you can use the definition of sine of the acute angles of a right triangle. Consider the same triangle as in the previous step. The sine of the angle of inclination of apogamy to the base is by definition equal to the ratio of the length of the opposite leg (height of pyramid) to the hypotenuse (the apofema). This implies that for the calculation of a desired value it is sufficient to multiply the apofema of the sine of the angle: H = h*sin(α).