You will need
- - drawing utensils;
- - a notebook in a cage;
- - the theorem of sines;
- - Pythagorean theorem;
- calculator.
Instruction
1
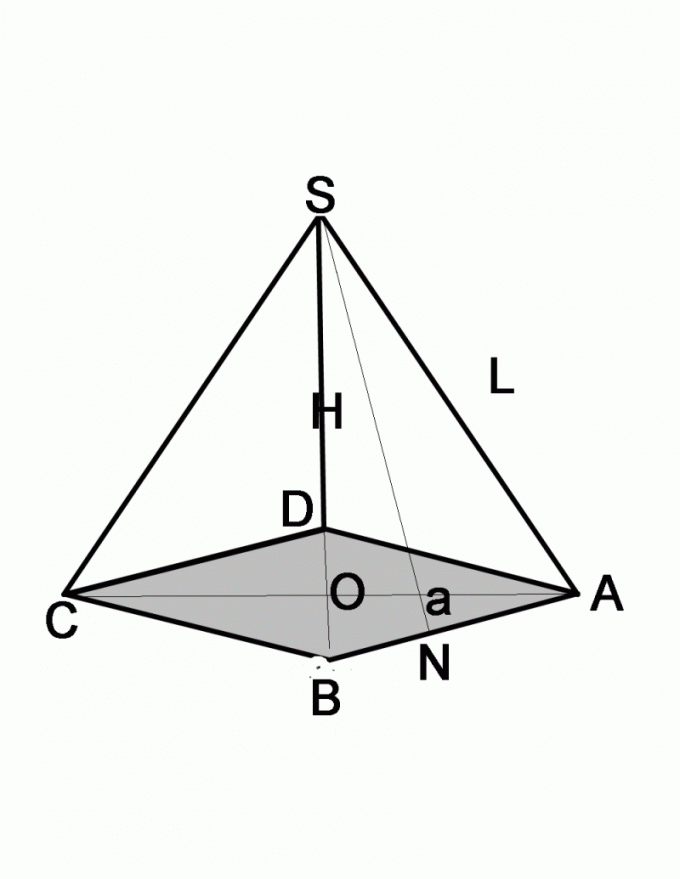
In the school geometry course focuses mostly on the pyramid, at the base of which is a regular polygon, that is, one in which all sides are equal. The projection of the vertex of the pyramid coincides with the center of its base. Draw a pyramid, the base of which is an equilateral triangle. The conditions can be given:
- the length of the lateral edges of the pyramid and its angle with the edge between the side face and the base;
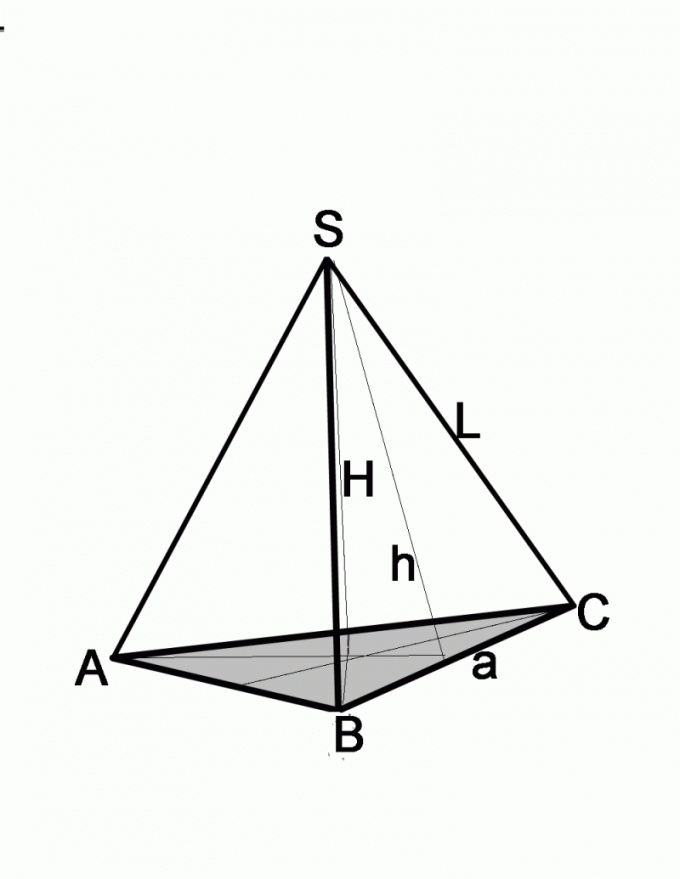
- the length of the lateral edges and the height of the side faces;
- the length of the lateral edges and the height of the pyramid.
- the length of the lateral edges of the pyramid and its angle with the edge between the side face and the base;
- the length of the lateral edges and the height of the side faces;
- the length of the lateral edges and the height of the pyramid.
2
If you know the lateral edge and corner, the problem is solved somewhat differently. Remember, what constitutes each lateral face of a pyramid, the base of which lies an equilateral polygon. This is an isosceles triangle. Swipe its height, which is also the angle bisector and median. That is half the base a/2=L*cosA, where a is side of base of the pyramid, L is the length of the edge. To find the size of the base, enough the result multiplied by 2.
3
If the task given the height of the side edges, and edge length, find the base of the Pythagorean theorem. The side in this case is the hypotenuse, a known high –z one of the shorter sides. To find the length of the second leg from the square of the hypotenuse subtract the square of the second leg, that is (a/2)2=L2-h2, where a – side of the base, L is the side length of, h – height of the side faces.
4
In this case, you must perform additional build to be able to use trigonometric functions. You are given lateral edge L and the height of the pyramid H, which connects the top of the pyramid with the center of the base. From the point of intersection of the altitude with the plane of the base guide segment connecting this point with one of the corners of the base. You got a right triangle, the hypotenuse of which is the lateral edge, one of the sides – the height of the pyramid. According to these data it is easy to find the second leg of the triangle, it is enough out of square side edges L subtract the square of the height H. Further actions depend on whether a particular figure is the Foundation.
5
Summarize the properties of an equilateral triangle. His height are both bisectors and medians. At the point of intersection, they are divided in half. So you found a half the height of the base. For computational convenience spend all three heights. You will see that cut, the square of the length of which you already found, is the hypotenuse of a right triangle. Extract the square root. You know, and the acute angle is 30°, so to find half of the base will not be difficult, applying the theorem of cosines.
6
For the pyramid, the base of which lies the right quadrilateral, the algorithm will be the same. If you subtract the square of a side edge of the square of the height of the pyramid, get the squared half the diagonal of the base. Extract the root, find the diagonal size, which is also the hypotenuse of an isosceles right triangle. The size of any of the other two sides look at the Pythagorean theorem, law of sines or cosines.