Instruction
1
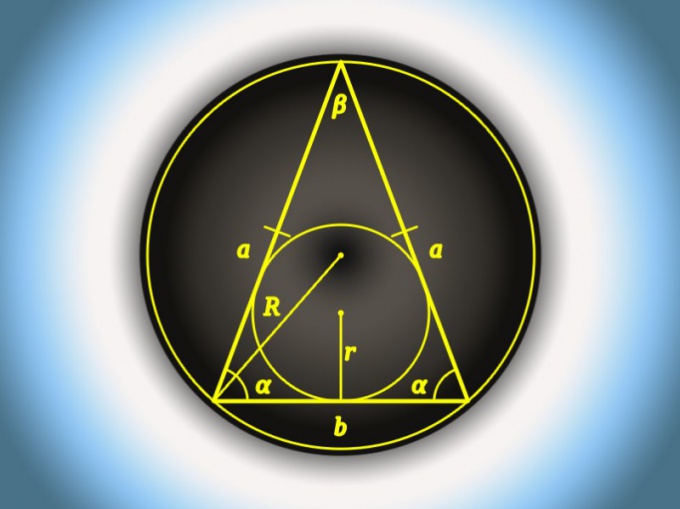
The length of the base (b) isosceles triangle in which the length of the lateral side (a) and the magnitude of the base angle (α), calculate using the theorem of projections. From this it follows that the desired value is equal to two lengths of the sides multiplied by the cosine of the known values: b = 2*a*cos(α).
2
If the conditions the previous step to replace the angle adjacent to the base, the angle lying opposite to it (β), calculate the length of this side (b) you can use the size of the sides (a) and the other trigonometric function is the sine of half the angle. These two values multiply and double: b = 2*a*sin(β/2).
3
For the same initial data as in the previous step, there is another formula, but it also trigonometric functions includes the root. If you are not afraid, subtract from unity the cosine of the angle at the vertex of the triangle double the value obtained, extract from the root of the result and multiply it by the length of the sides: b = a*√(2*(1-cos(β)).
4
Knowing the length of the perimeter (P) and lateral side (a) of an isosceles triangle to find the length of base (b) is very easy - just subtract the first two values of the second: b = P-2*a.
5
The area value (S) of such a triangle is also possible to calculate the length of the base (b), if the known height (h) of the figure. This double square is divide by the height: b = 2*S/h.
6
Height (h) is omitted on the basis of (b) an isosceles triangle can be used to calculate the length of this side in combination with the length of the sides (a). If these two parameters are known, the lift height in the square, subtract from the obtained values of the squared lengths of the sides, from the result extract the square root, and double: b = 2*√(h2-a2).
7
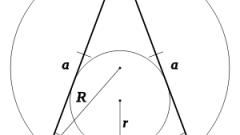
Can be used to calculate the length of the base (b) and the radius (R) of a triangle circumscribed about a circle if you know the angle lying opposite the base (β). Deuce multiply by the radius and the sine of that angle: b = 2*R*sin(β).