Instruction
1
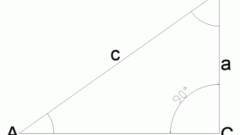
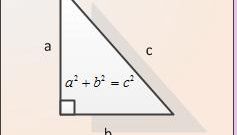
School classic formulation of the Pythagorean theorem is: the square of the hypotenuse is equal to the sum of the squares of the other two sides. Thus, to find the hypotenuse of a right triangle two legs, alternately, need to square the lengths of the legs, fold them and take the square root of the result. In its original formulation, the theorem asserted that the area of a square constructed on the hypotenuse equal to the sum of the areas of two squares constructed on the legs. However, modern algebraic formulation does not require to introduce the concept of square.
2
For example, given a right triangle, the legs of which is equal to 7 cm and 8 cm Then, according to the Pythagorean theorem, the square of the hypotenuse is 72+82=49+64=113 cm2. Itself the hypotenuse is equal to the square root of the number 113. Happened irrational number, which goes in the answer.
3
If the sides of a triangle are 3 and 4 then the hypotenuse equals √25=5. When extracting the square root turned out to be a natural number. Numbers 3, 4, 5 are a Pythagorean triple because they satisfy the condition x2+y2=z2, being all natural. Other examples of Pythagorean triples: 6, 8, 10; 5, 12, 13; 15, 20, 25; 9, 40, 41.
4
If the legs are equal, then the Pythagorean theorem into a more simple equation. Suppose, for example, both sides equal to the number A, and the hypotenuse is designated for C. Then C2=A2+A2 C2=2A2, C=A√2. In this case, no need to build in the square of the number A.
5
The Pythagorean theorem is a special case of a more General theorem of cosines, which sets the ratio between the three sides of the triangle for an arbitrary angle between any two of them.
Useful advice
Right triangle, whose sides relate as 3:4:5 called Egyptian triangle, since such figures were widely used by the architects of Ancient Egypt. It is also the simplest example of Garanovich of triangles in which the sides and the area represented by integers.