How to find the derivative of given function

The task of taking the derivative of the given function is the base for secondary school students, and for students of higher educational institutions. Is impossible to learn mathematics without learning the concept of derivative. But do not worry ahead of time - any derivative can be calculated using simple algorithms of differentiation and knowing derivatives of elementary functions.

You will need

• Table of derivatives of elementary functions, rules of differentiation

Instruction

1. By definition, the derivative of the function is the ratio of the increment function to increment of argument for an infinitely small period of time. Thus, the derivative shows the dependence of the growth function from changing the argument.
2. In order to find the derivative of elementary functions is enough to use a table of derivatives. Full table of derivatives of elementary functions are shown in Fig.
   
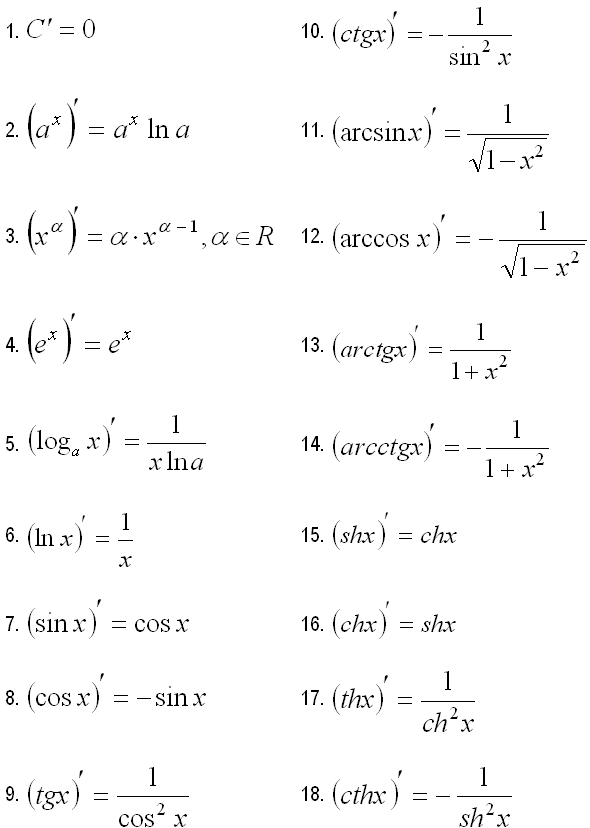
3. In order to find the derivative of a sum (difference) of two elementary functions, we use the rule for differentiating sums: the derivative of sum of functions is equal to the sum of their derivatives. It is written as:
   
   (f(x)+g(x))'=f'(x)+g'(x). Here the symbol (') is shown taking the derivative of the function. And then the problem reduces to taking derivatives of the two basic functions described in the previous step.
4. In order to find the derivative of product of two functions, it is necessary to use one more rule of differentiation:
   
   (f(x)*g(x))'=f'(x)*g(x)+f(x)*g'(x), that is, derivative works equal to the sum of the product of the derivative of the first multiplier to the second and first multiplier on the derivative of the second. To find the derivative of the private by the formula shown in the picture. It is very similar to the rule of taking the derivative works, only instead of the sum in the numerator is the difference and is added to the denominator, which is the square of the denominator of the given function.
   
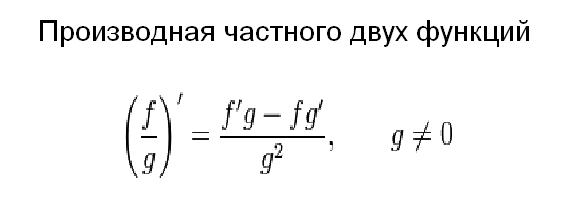
5. Taking the derivative of a composite function is the most difficult task while differentiation of (complex function is called the function argument which is any dependency). But she decided on a fairly simple algorithm. First we take the derivative for complex argument, believing it to be simple. Then we multiply the obtained expression for the derivative of complex argument. So we can find the derivative of the function with any degree of nesting.