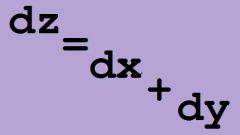Instruction
1
In the first place in order to learn how to solve limits, you need to understand what is the limit. This concept means that some variable that depends on some other value approaches a specific value as the second change of this magnitude. The limit is denoted lim sign (x). Under this sign indicated aspires to H. If it is specified, for example, x>5, then it shows that the value of x is constantly striving to five. The entry reads as "the limit of the function when x approaches five. Now there are a huge number of ways to solve limits.
2
In order to better understand how to solve limits, you need to parse the following example. Let's say this: lim as x>2=3-4/x+3. First, try to understand, SBA that implied that "x approaches two. This expression means that x eventually changes their values. But these values every time are closer and closer to the value equal to two. In other words, it is 2.1, then 2.01, but 2,001, 2,0001, 2,00001. And so on to infinity.
3
From the foregoing, it is possible to make a definite conclusion that x is numerically almost identical with the value equal to two. On this basis, this example is easy to solve. You just need to substitute the two given function. Get: 3*2-4/2+3=6-2+3=7.
Useful advice
At the solution of a limit first, always try to substitute the number sought by x in the function. If x is not committed to a specific number and to infinity, then the limit is infinite. If you doubt the correctness of his decision, it does not matter. There are online services that are designed to solve limits. For example, on the website http://www.kontrolnaya-rabota.ru/s/predel/funktsii/ to solve the limit? you just need to enter all your data into a convenient form. The program will consider everything and give the answer.