You will need
- Compasses, pencil, sheet of paper
Instruction
1
Take a sheet of paper and put it in the middle point O. It will be the center of the circle. Set the distance of the legs of a compass equal to the radius of the circle. Swipe a circle with a specified radius.
2
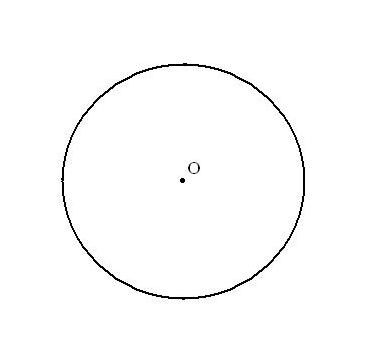
Anywhere on the arc of a circle place the point M. It will enter the first vertex of a Pentagon. Through the point M and the guide diameter of the circle MN. To build a straight line, use any available object with a flat side.
3
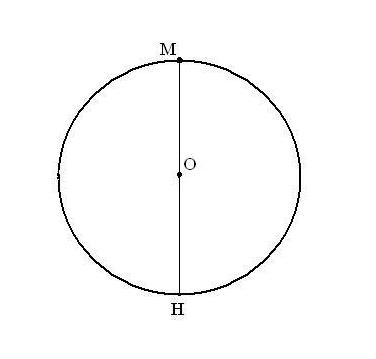
Construct another diameter perpendicular to the diameter MN. To do this, swipe the compass arcs from the points M and N with the same radius. Select the radius such that both arc crossed among themselves and with the circumferenceu at the same point. This will be the first point And the second diameter. Swipe through it and point On the line. Get the diameter AB, the perpendicular line PL.

4
Find the center of the radius. For this purpose a compass with the radius of the circle, slide the arc from point b so that it crosses the circle in two points s and R. Through these points draw a straight. This video will divide the radius AT exactly half. Put a point in the intersection of the SR and IN.
5
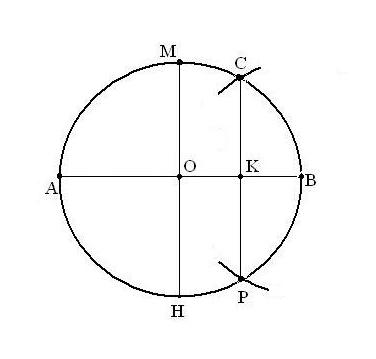
Connect the point M To the line segment. Set on the compass a distance equal to the segment MK. From point M draw a arc so that it crosses the radius AO. In place of this crossing you have to put the distance IU is equivalent to the length of one side enter the Pentagon.

6
Build the remaining vertices of a Pentagon. To do this, set the distance of the legs of a compass equal to segment ME. From the first vertex of the Pentagon M swipe an arc to intersection with the circleYu. The point of intersection will be the second vertex Of F. the points obtained in turn also swipe an arc of the same radius with the intersection of the circle. Get the third vertex of the Pentagon G. similarly construct the remaining points S and L.
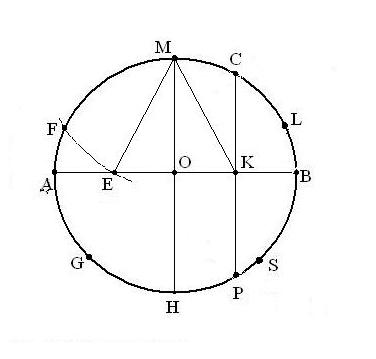
7
Connect the resulting vertices straight lines. Inscribed in a circle, Pentagon MFGSL built.