How to find the side of a regular hexagon

Hexagonal - the "hexagonal" form, for example, have a section of nuts and pencils, honeycomb and snowflakes. A regular geometric shape, such shapes have certain features that distinguish them from other flat polygons. It consists in the fact that the radius of the hexagon described about a circle equal to the length of its sides, in many cases, this greatly simplifies the calculation of the parameters of the polygon.

How to find the side of a regular hexagon
How to find the area of a regular hexagon
How to find the sides of a polygon
How to find the area of the hexagon

Instruction

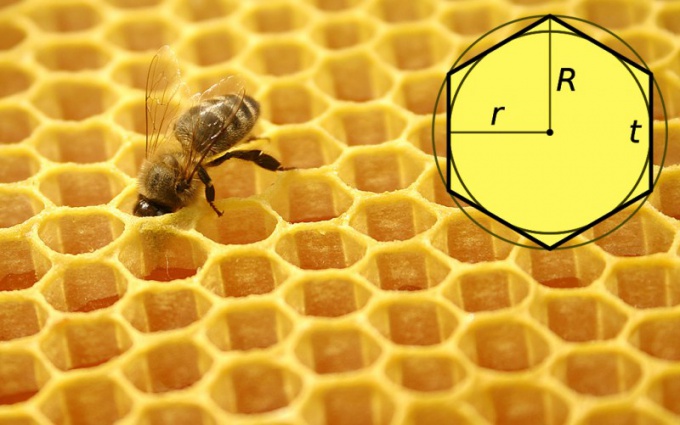
If the conditions of the problem given the radius (R) described about the right of the hexagon of a circle, calculate do not have - this value is identical with the length of the side (t) of the hexagon: t = R. in the case of known diameter (D) simply divide it in half: t = D/2.

The perimeter (P) the right of the hexagon allows us to calculate the length of a side (t) by a simple operation of division. As divider use the number of parties, i.e. six: t = P/6.

The radius (r) inscribed in a polygon circle is associated with the length of its side (t) is slightly more complex ratio - double the radius, and the result divide by the square root of triples: t = 2*r/√3. The same formula using the diameter (d) of the inscribed circle will be one mathematical operation in short: t = d/√3. For example, if the radius of 50 cm side length of the hexagon should be approximately equal to 2*50/√3 ≈ 57,735 see

Known area (S) of a polygon with six vertices also allows us to calculate the length of its side (t), but the numerical factor linking them, just expressed through the fraction of three natural numbers. Two thirds of the area divide by the square root of three, and the obtained values remove the square root: t = √(2*S/(3*√3)). For example, if the area of the figure is 400 cm2, the length of her hand should be about √(2*400/(3*√3)) ≈ √(800/5,196) ≈ √153,965 ≈ 12,408 see

The circumference (L) described around the right hexagon, connected with the radius and, therefore, with side length (t) through the number PI. If it is given in terms of the problem, divide its value by two PI is: t = L/(2*π). For example, if this value is equal to 400 cm, side length should be approximately 400/(2*3,142) = 400/6,284 ≈ 63,654 see

This is the same parameter (l) for the inscribed circle allows you to calculate the length of a side of the hexagon (t) by calculating the ratio between it and the product of the number PI by the square root of triples: t = l/(π*√3). For example, if the length of the inscribed circle is 300 cm, the side of the hexagon needs to have a value approximately equal to 300/(3,142*√3) ≈ 300/(3,142*1,732) ≈ 300/5,442 ≈ 55,127 see

Is the advice useful?