How to find the length of the hypotenuse in a right triangle

Call the hypotenuse the longest side in a right triangle, therefore, not surprising that in the Greek language this word is translated as "stretched". This side is always opposite the angle to 90° and the sides that form the angle are called the legs. Knowing the lengths of these sides and the magnitude of the acute angles in different combinations of these values, we can calculate the length of the hypotenuse.

Instruction

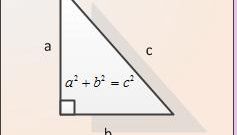
1. If you know the lengths of both legs of the triangle (A and b), then use to find the length of the hypotenuse (C) is probably the most famous on the planet a mathematical postulate - the Pythagorean theorem. It States that the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the legs, from which it follows that you should calculate the square root of the sum of squared lengths of two known sides: C=√(A2+B2). For example, if the length of one leg is 15 inches and the other 10 centimeters, the length of the hypotenuse will be approximately 18,0277564 centimeters, as √(152+102)=√(225+100)= √325≈18,0277564.
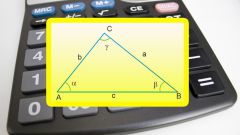
2. If you know the length of only one of the legs (A) in a right triangle, and the angle lying opposite it (α), the length of the hypotenuse (C) can be determined using one of the trigonometric functions - sine. To do this, divide the length of the known side by the sine of the known angle: C=A/sin(α). For example, if the length of one of the legs is equal to 15 centimeters and the angle at the opposite vertex of the triangle is 30°, the length of the hypotenuse is equal to 30 centimeters, as 15/sin(30°)=15/0,5=30.
3. If in a right triangle, we know the value of one of the acute angles (α) and the length of the adjacent leg (B), to calculate the length of the hypotenuse (C) you can use another trigonometric function, the cosine. You should divide the length of the known leg to the cosine of the known angle: S=V/ cos(α). For example, if the length of this side is 15 centimeters and the magnitude of the acute angle, the Annex, is 30°, the length of the hypotenuse will be approximately 17,3205081 centimeters, as 15/cos(30°)=15/(0,5*√3)=30/√3≈17,3205081.