By EasyHow

The diameter of a circle: how to define it

With a circumference associated with many interesting, beautiful and difficult theorems in geometry. Our task is one of the most simple: we have to find diameter of a circle. Will try to do it using two formulas.

The diameter of a circle: how to define it
How to find the diameter of a circle
How to determine the diameter of a circle
How to measure the diameter of the circle

Instruction

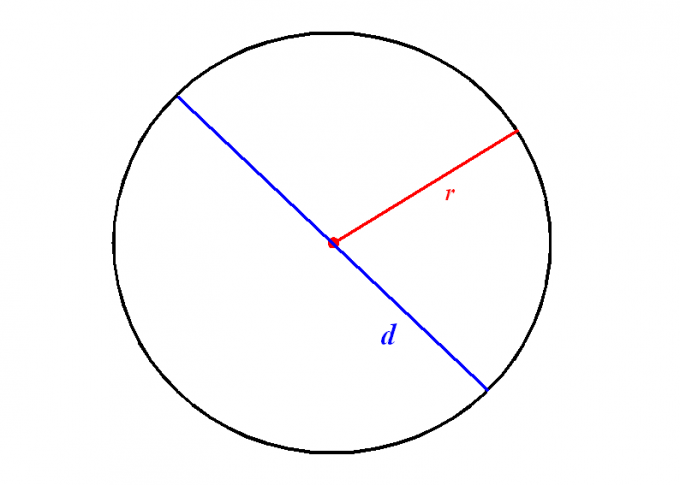
A segment that connects any two points of a circle is called a chord.Passing through the center of a circle, the chord is called its diameter. The diameter denoted by the symbol  or Latin letter D. Diameter (D) twice as long as the radius of curvature (R) and is the greatest possible distance between the points on the circle.Example. The radius of the circle is 20 cm D(diameter)? Then, if R = 20 cm and we know that the length of the diameter equal to the length of the two radii D = 2R = 2*20 = 40 cm

There is a second way to find the diameter of a circle. In this case, we should be known for its length. Mark the circumference of the Latin letter C. Example. C = 60 cm D - ? Solution. From geometry we know that the circumference is given by: C = 2R, where: R is the radius of the circle, and  is an irrational number "PI" equal approximately to 3.14. Then, this formula implies the other: D = With : 3,14. So, D = 60 : 3,14 = 19,12 see

Useful advice

A circle is sometimes called the "wheel geometry". One of the properties of the wheel, its axis remains all the time at a constant distance from the surface on which it rolls in the mathematical formulation becomes the definition of the circle. Circle is the set of points in the plane, distant from a fixed point, its centre, the same distance, or radius (from Latin radius "spoke wheels", "ray"). Radii are also called line segments connecting the center points of the circle.

The diameter divides the circumference into equal parts. Commenter "Started" the ancient Greek philosopher Proclus, who lived in the V century, attributed this discovery to Thales, the acknowledged founder of ancient philosophy and science. Clarifying this statement, we can say that a circle symmetric about any diameter (or rather, its containing straight).*

* Encyclopedia for children, volume 11 of Mathematics, Moscow, "AVANTA+", 1998.