Instruction
1
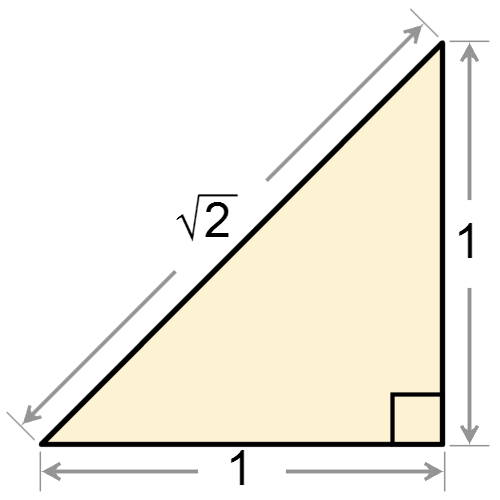
From the name of a right triangle it is clear that one of its angles is a direct. Regardless of whether it is an isosceles right triangle or not, it always has one angle equal to 90 degrees. If given a right triangle that is both isosceles and then, based on the fact that the figure is a straight angle, find two angles at its base. These angles are equal, so each of them has a value equal to:
α=180°- 90°/2=45°
α=180°- 90°/2=45°
2
In addition to the above, it is also possible the other case, when the triangle is rectangular, but is not isosceles. In many problems the angle of the triangle is 30° and the other 60°, because the sum of all angles in a triangle must equal 180°. If given the hypotenuse of a right triangle and side angle can be found from matching the two sides:
sin α=a/c where a is the side opposite to the hypotenuse of a triangle, with the hypotenuse of the triangle
Accordingly, α=arcsin(a/c)
Also the angle you can find the formula for cosine:
cos α=b/c where b is the adjacent side to the hypotenuse of the triangle
sin α=a/c where a is the side opposite to the hypotenuse of a triangle, with the hypotenuse of the triangle
Accordingly, α=arcsin(a/c)
Also the angle you can find the formula for cosine:
cos α=b/c where b is the adjacent side to the hypotenuse of the triangle
3
If you know only two sides, the angle α can be found according to the formula of the tangent. The tangent of this angle is equal to the ratio of opposite over adjacent:
tg α=a/b
From this it follows that α=arctg(a/b)
When the straight angle and one of the corners found by the above method, the second is as follows:
ß=180°-(90°+α)
tg α=a/b
From this it follows that α=arctg(a/b)
When the straight angle and one of the corners found by the above method, the second is as follows:
ß=180°-(90°+α)