Instruction
1
The most common method to measure an average value in the range of values is the arithmetic mean. In order to calculate it, you need the sum of all values of a series divided by the number of these values. For example, if given a number 3, 4, 8, 12, 17, its arithmetic mean is equal to (3 + 4 + 8 + 12 + 17)/5 = 44/5 = 8,6.
2
Another average commonly encountered in mathematical and statistical tasks, called harmonic mean. Harmonic mean of the numbers a0, a1, a2... an is equal to n/(1/a0 + 1/a1 + 1/a2... +1/an). For example, the same numberas in the previous example, the harmonic mean will be equal 5/(1/3 + 1/4 + 1/8 + 1/12 + 1/17) = 5/(347/408) = 5,87. Harmonic mean is always less than the arithmetic mean.
3
Different medium are used in different types of tasks. For example, if you know that the first hour the car was traveling at a speed A, and the second — with speeds of B, then its average speed during the journey will be the average between A and B. But if you know that the car traveled one kilometer at a speed A, and the next with the speed B, then to calculate his average speed during the journey, you will need to take the harmonic mean between A and B.
4
For statistical purposes, the arithmetic mean is a convenient and objective evaluation, but only in those cases when the values of a number not dramatically eye-catching. For example, the number of 1, 2, 3, 4, 5, 6, 7, 8, 9, 200 the arithmetic mean is equal to 24, 5 — much more of all the members of the seriesexcept the last one. It is obvious that such an estimate cannot be considered fully adequate.
5
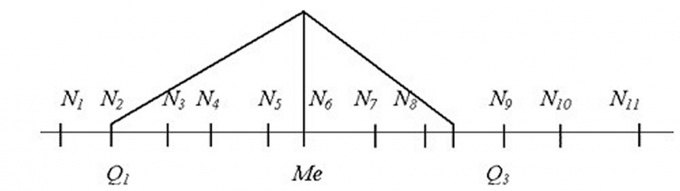
In such cases, you must calculate the median of a number. This average value, the value of which is exactly in the middle of the row so that all members of the serieslocated before the median — not more than it, and all that comes after — not less. Of course, you need to first arrange the members of a number in ascending order.
6
If the row a0,... an odd number of values, i.e. n = 2k + 1, then the median is taken by a member of a number of with a serial number k + 1. If the number of values is even, i.e. n = 2k, then median is considered to be the arithmetic mean of the members of a number of numbers k and k + 1.
For example, already considered a number of 1, 2, 3, 4, 5, 6, 7, 8, 9, 200 ten members. Consequently, the median is the arithmetic mean between the fifth and sixth members, that is (5 + 6)/2 = 5,5. This estimate is much better reflects the average value of a typical member of the series.
For example, already considered a number of 1, 2, 3, 4, 5, 6, 7, 8, 9, 200 ten members. Consequently, the median is the arithmetic mean between the fifth and sixth members, that is (5 + 6)/2 = 5,5. This estimate is much better reflects the average value of a typical member of the series.