You will need
- calculator;
- paper;
- pencil.
Instruction
1
First, remember that a fraction is merely a conditional record dividing one number by another. Unlike addition and multiplication, when dividing two integers is not always possible integer. Here and agreed to call these two "dividing" number of the fraction. The number that is divided is called the numerator and that is divided is the denominator.
2
To write the fraction, write the numerator first, then slide under that number with a horizontal line and below the line write the denominator. The horizontal line dividing the numerator and denominator is called fraction bar. Sometimes it is portrayed as a slash "/" or "per". In this case, the numerator is written to the left from the line and the denominator on the right. So, for example, the fraction "two-thirds" will be written as 2/3. For clarity, the numerator is usually written at the top of the line and the denominator is at the bottom, that is, instead of 2/3 can be found: ⅔.
3
If the numerator more than its denominator, then this "wrong" fraction is usually written in the form of "mixed" fractions. In order to receive a mixed improper fraction, simply divide the numerator by the denominator and write down the resulting quotient. Then place the remainder in the numerator of the fraction and write the fraction to the right of the quotient (the denominator do not touch). For example, 7/3 = 2⅓.
4
To add two fractions with the same denominator, simply add their numerators (the denominators do not touch). For example, 2/7 + 3/7 = (2+3)/7 = 5/7. Similarly produce and subtraction of two fractions (numerators at the same time deducted). For example, 6/7 – 2/7 = (6-2)/7 = 4/7.
5
To add two fractions with different denominators, multiply the numerator and denominator of the first fraction by the denominator of the second, and the numerator and denominator of the second fraction – by denominator of the first. In the end you get the sum of two fractions with the same denominators, addition of which is described in the previous paragraph.
For example, 3/4 + 2/3 = (3*3)/(4*3) + (2*4)/(3*4) = 9/12 + 8/12 = (9+8)/12 = 17/12 = 1 5/12.
For example, 3/4 + 2/3 = (3*3)/(4*3) + (2*4)/(3*4) = 9/12 + 8/12 = (9+8)/12 = 17/12 = 1 5/12.
6
If the denominators of the fractions have a common divisor, that is divided by the same number, then select as common denominator the smallest number divisible by the first and second denominator at the same time. For example, if the first denominator is 6 and the second 8 as a common denominator not take their work (48), and the number 24 which is split as at 6, and 8. The numerators of the fractions in this case are multiplied by the quotient of the common denominator by the denominator of each fraction. For example, for the denominator, 6 in this number will be 4 (24/6), and for the denominator 8 – 3 (24/8). More clearly, this process is visible in a specific example:
5/6 + 3/8 = (5*4)/24 + (3*3)/24 = 20/24 + 9/24 = 29/24 = 1 5/24.
Subtraction of fractions with different denominators is quite similar.
5/6 + 3/8 = (5*4)/24 + (3*3)/24 = 20/24 + 9/24 = 29/24 = 1 5/24.
Subtraction of fractions with different denominators is quite similar.
7
To multiply two fractions, multiply between their numerators and denominators.
For example, 2/3 * 4/5 = (2*4)/(3*5) = 8/15.
For example, 2/3 * 4/5 = (2*4)/(3*5) = 8/15.
8
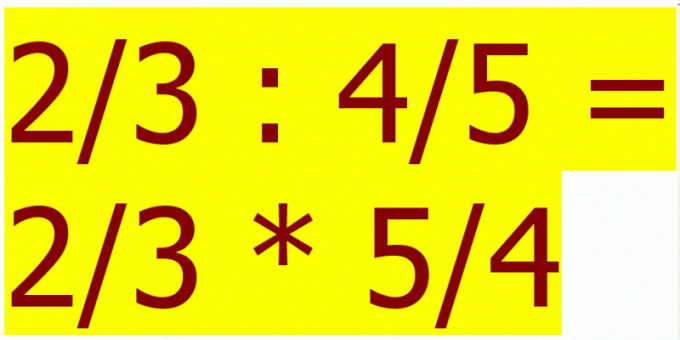
To divide two fractions, multiply the first fraction by the inverted (reverse) the second fraction.
For example, 2/3 : 4/5 = 2/3 * 5/4 = 10/12.
For example, 2/3 : 4/5 = 2/3 * 5/4 = 10/12.
9
To reduce a fraction, divide its numerator and denominator by the same number. For example, the result of the previous example (10/12) can be written as 5/6:
10/12 = (10:2)/(12:2) = 5/6.
10/12 = (10:2)/(12:2) = 5/6.